The puzzle-gaming genre of “making shapes out of formulas” is currently ruled by a single designer. Five years after his last sensational stab at the genre, Iranian-born game-maker Mahdi Bahrami has returned with an even more impressive—and, at times, hair-pullingly difficult—puzzling masterpiece.

Tandis, out this week on both Steam and as a direct, DRM-free purchase for $15 (temporarily on sale for $13.49), is arguably the coolest execution of high-level math I’ve ever seen in a PC game. Even better, its challenge is disguised in the form of a tinker-toy. Hand this to any young, budding mathematician, and watch them get hooked on what’s ultimately a brilliant edutainment gem in disguise.

Axes and allies

The beauty of Tandis comes from how it turns the formulaic manipulation of X, Y, and Z axes into a gaming mechanic. Typical education about mathematical formulas revolves around plotting solution results on a 2D grid to see what shapes they generate. That’s fine enough—though doing this requires the mathematical grokking of a formula itself. But what if you could do this kind of thing much faster, and in 3D, by dragging shapes onto an easily understood series of grids, then watching them transform into fantastic new shapes in response?

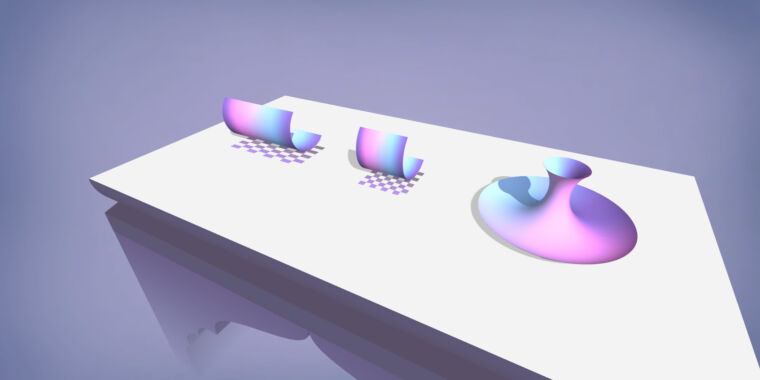
In every Tandis puzzle, players are handed a single geometric shape (sometimes with full 3D properties, sometimes as a flat 2D polygon), then are shown the puzzle’s solution, which is a different geometric shape. Let’s start with a simple example. In the game’s first puzzle, your starting shape (which you can pick up) is one-fourth the volume of the “solution” block (which you cannot touch).

Pick the shape up with your mouse, then place it on a grid made of black-and-white squares. To the right of that, a new shape appears on a grid of bigger black-and-white squares, and the resulting shape is twice as large on all axes—which, coincidentally enough, is how much bigger the right-side black-and-white squares are. (Mathematically, that’s a simple multiplication of all axes’ values.) You can pick up either shape, and at this point, the larger one perfectly matches the solution shape. Pick it up and move it to a podium next to the solution and Tandis will scan your submission to confirm that its size, shape, and curves are close enough.

The next puzzle hints to how hairy Tandis will eventually become. Its left-side grid is made of black-and-white squares, while the other grid is full of squiggly lines. Put a right-angled shape onto the left-side grid, and it will come out of the right-side grid with its X and Y axes in equally squiggly form. (Mathematically, we’re looking at a parabolic equation applied to a single axis here.)

From here on out, Tandis only becomes more intense, and its challenge potential is perhaps best summarized by the below collection of shape-manipulation GIFs, as made by Bahrami.

If a puzzle has multiple grids on its table, you can put a shape onto any of them to generate manipulated shapes on the other grids. Use your mouse to lift any one of those new shapes, then move it onto another grid for the sake of a recursive formula application. If one grid transforms a 2D shape into a 3D donut, you can grab the resulting donut, shift it onto the donut-izing grid, and distort it that much more as a result. The exact placement of 3D shapes onto the more intense grids additionally changes which portions of their shapes are manipulated.